Let’s continue to find and extract two more two-dimensional Platonic solids from Metatron’s cube and turn them into three-dimensional images. When we visualize Metatron’s cube as a three-dimensional image, we can begin to understand the motion within the cube that gives rise to the energy that it creates.
Once again, here is the traditional two-dimensional image of Metatron’s cube. The next Platonic solid that we want to focus on is the hexahedron or cube. Several squares are visible. Each square is a face of the cube. The cube is drawn in three-point perspective, so it is easy to see more than one face of the cube at a time.
There are two cubes within Metatron’s cube and if you were to extract both of them, you would have a tesseract. I will discuss the tesseract in greater detail in a subsequent blog. Click on the video below which shows the larger hexahedron morphing into a three-dimensional image.
The three-dimensional model that we are working to build is shown below. The three-dimensional hexahedron is shown in green. There are three faces of the hexahedron that are visible.
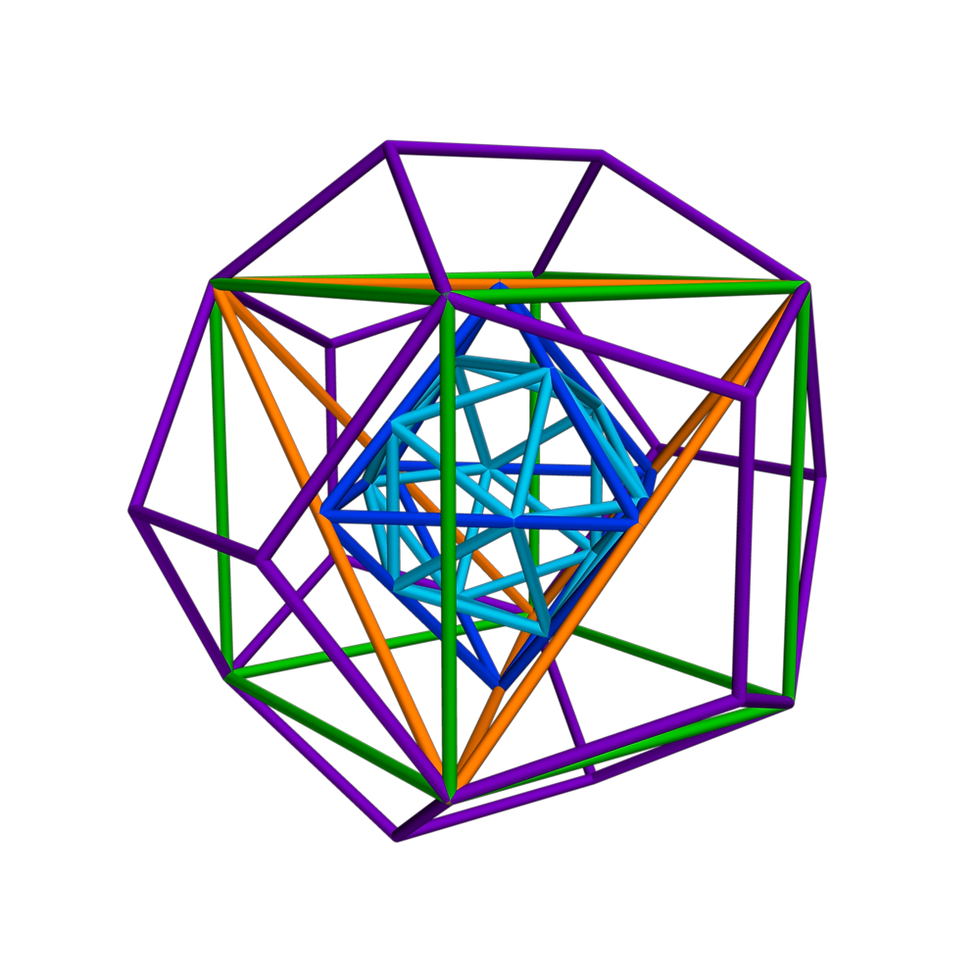
Let’s move on to the octahedron. There are at least two octahedrons in the two-dimensional Metatron’s cube and they can be visualized from different perspectives. We will focus on the big one that is visible looking at the cube straight on. Each face of the octahedron is a triangle. Click on the video below which shows the octahedron morphing into its three-dimensional shape.
When you have viewed the octahedron video, look again at the three-dimensional model above and you will see the blue octahedron in the middle of the figure. Only two faces are visible.
There is one more video to watch of the dodecahedron and the icosahedron and they will be presented in the next blog.
Comentários