This is the first in a new series of blogs about sacred geometry, projective geometry, and in particular Metatron's Cube. About 15 years ago, my partner and I started doing sessions with a team of entities who refer to themselves as “Our Team”. These entities exist in a different dimension, a dimension that they tell us is our true home. Sometimes they speak as a single voice and sometimes they speak with one voice.
About a year and a half ago we began a new series of conversations with the Team. We don't have a fixed agenda for these sessions. We basically listen to what they have to say and then have an opportunity for questions and answers. After several of these sessions, they shared information with us about Metatron’s Cube. This was the first time they had ever discussed sacred geometry.
In this series of blogs, I'll be sharing that information as best as I understand it with you. So, let's begin.
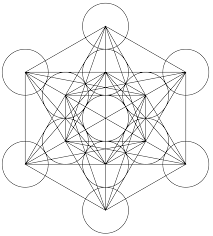
The first piece of information that they shared with us was that Metatron’s Cube is not a two-dimensional flat image. Indeed, it would be inaccurate to describe it as solely a three-dimensional construct. But let's start with the three-dimensional aspects of it.
If you are anything like me, you are familiar with the image of Metatron's Cube that is shared here. And you can see that it is clearly two-dimensional. However, if you look at it closely enough, you can see that there are some shapes inside the cube that are drawn with a two-point perspective. Some of the figures appear to be 3D even though they are on a two-dimensional piece of paper.
One of the best examples is the cube that is in the center of the image. You can trace out the edges of the cube and see all six sides. If you were to shade in the sides you would see a top side and a left side and a right side. The bottom side and the two rear sides would not be visible.
Let’s start with some basic definitions of the shapes inside Metatron’s Cube and I'm not going to get too technical here. Metatron’s Cube contains the five Platonic solids. They're called Platonic solids in honor of the philosopher Plato who seems to have been the earliest one to write them down and teach about them.
The platonic solids are comprised of five regular polygons, convex in nature. Polygons are two dimensional shapes comprised of straight lines. Convex means all interior angles are less than 180 degrees. Think of a triangle. A regular polygon has all sides of the same length and all angles equal. Think of a square. But a triangle can also be a regular polygon.
A polyhedron is a three dimensional polygon. So, when the square becomes a cube, the cube is a polyhedron. The Platonic solids are also the only regular, convex polyhedra. The remaining three Platonic solids are the octahedron which is eight-sided, the dodecahedron which is twelve-sided, and the icosahedron which is twenty-sided.

Consider what this image might look like in three dimensions. And to give you
an idea of what I'm talking about look at this next picture which shows a three-dimensional portion of Metatron's Cube projected onto a wall and you will notice the familiar Metatron’s Cube image. This picture demonstrates dimensionality. And keep in mind that the third dimensional image is just a portion of Metatron's Cube.

So, what does a three-dimensional Metatron’s Cube look like? This next picture is a very interesting representation of Metatron’s Cube in 3D. As you can see there's a lot going on there. Seeing Metatron’s Cube as a three-dimensional shape gives a fuller understanding of the complexity of it. And seeing all the shapes in motion would almost bring it to life. For now, we will just have to imagine what that would look like.
This first blog is all about perspective. When you take something familiar, the image of Metatron’s Cube, and expand it by adding a new perspective, in this case an extra dimension, it becomes more robust and easier to understand. It is also a closer representation of the true nature of Metatron’s Cube.
Comments