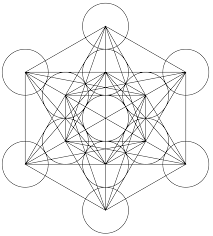
As we move deeper into Metatron’s cube and identify the Platonic solids that lie within, we begin to understand the marvelous complexity that is found there. And as we discuss the movement of these polyhedrons, our understanding of Metatron’s cube begins to shift from the motionless, two dimensional familiar image of Metatron’s cube to the robust and vibrant three dimensional image that more closely resembles the true nature of Metatron’s cube.
Let’s dive in and continue identifying the Platonic solids within the two dimensional image of Metatron’s cube. The next shape that I find easy to identify is the cube or hexahedron. The hexahedron is a six-sided polyhedron, and it is easy to see right in the middle of Metatron’s cube. There are actually two hexahedrons in Metatron’s cube. Can you find the other one? I’ll give you a hint. It is bigger than the one in the middle.

I mentioned a little bit about perspective in the last blog. In the first image above, you can see that the cube is drawn in two point perspective so you can see all six sides. In this next image, we have shaded three of the sides, the top and the two front sides. The other sides, the bottom and the back sides, are now somewhat obscured. It may be easier to see the larger hexahedron in this second image.

We are going to look at one more Platonic solid and that is the octahedron which has eight sides. This one is a little harder to find. And I want to mention that there are two octahedrons also. You can only see four sides of the larger octahedron. In this next picture we have shaded four sides of the smaller octahedron. If you have a good imagination you might be able to see one or two of the other sides.
If you are having trouble seeing the larger octahedron, start with the original large triangle that is facing front and connects to the top circle. Then just connect the other outside circles with straight lines and the other three sides should become visible.
Now that we've identified the two hexahedron's and the two octahedrons in Metatron’s cube, I'd like you to visualize the larger cube and the smaller octahedron together. Visualize the octahedron inside of the larger cube.

Now take a look at this next image and you'll see a 3D piece of jewelry with the octahedron inside the hexahedron. You'll see that it is actually being held it a person's hand. And at the same time a light is shining on it. The reflection appears on the surface behind it which is of course a 2 dimensional structure. I hope you can see that the image on the surface is our familiar image of Metatron's cube.
When I first saw this the picture, I thought what's to the left, that is to say what's in a higher dimension than the third dimension that is creating the three dimensional image? And that's a discussion for another day. But focusing on the two dimensional representation on the wall, I hope you can understand now why I put so much emphasis on Metatron’s cube being a 3 dimensional object.
I promise to keep this next part easy to follow. Regular geometry, Euclidean geometry, is concerned with the measurement of length and angles. There is a special type of geometry called projective geometry which has to do with projection, as the above example demonstrates, and it does not preserve length and angle. It does however preserve many properties and it creates some new ones.
One of the principles that is new to projective geometry is that of polarity. Another new principle is duality. I’m not going to attempt to define what these terms mean here. I will just make that statement that the hexahedron and the octahedron are duals of each other. The hexahedron has 3 edges per vertex and four edges per face and the octahedron has 4 edges per vertex and 3 edges per face. Remember, a vertex is where the edges come together. A face is what I have referred to as a side.

Imagine an octahedron inside of a hexahedron. Each octahedron vertex touches the hexahedron right in the middle of each face. And if you were to switch the two around so that the hexahedron is inside the octahedron, each hexahedron vertex would touch the octahedron right in the middle of each of its faces. I find that to be pretty interesting. That’s all I want to mention about duals for the time being. Just try to get a clear image of their points touching the other in the middle of their faces. We will talk more about duals in the next blog.
Metatron’s cube is a building block for all that exists in our world. It is a template. We have only briefly discussed the movement and the energy contained within it. Before we dive more deeply into those concepts, there are two more Platonic solids for us to discover and we will do that in the next blog. We have only begun to scratch the surface of this key to creation.
Comments